Sag & Tension in transmission line
Today we are going to learn a topic called sag and tension in the transmission lines.During the erection of overhead transmission line, the conductors are connected between two tower structures. The conductors connected between the tower structures must not be connected too tight or too loose. If they are connected very tightly, the tension on the conductor will be very high and at some point it may break. If they are connected very loose, the charging current increases the length of wire to be used and the height of the tower structure increases. Hence, the conductors must be connected in such a way that the tension is minimum and at the same time there should be good clearance between the ground and the conductor.Definition of Sag In Transmission Lines
Sag can be defined as "The difference in level between the points of supports and the lowest point on the conductor is known as sag"
Hence ,sag determines the value of safe working tension and the minimum clearance of the conductor with respect to ground.The conductor sag should be kept to a minimum in order to reduce the conductor material required and to avoid extra pole height for sufficient clearance above ground level.
Factors Affecting Sag
The various factors which effects sag are,(i) Weight of conductor.
(ii) Location of conductor.
(iii) Length of span.
(iv) Temperature.
(v) Tensile strength.
(Vi) Tension.
Here we discuss briefly about various factors sag & tension in electrical transmission lines .
(i) Weight of conductor
The sag of an overhead line is directly proportional to the weight of the conductor.This is because the weight of any body acts vertically downwards.i.e.,more the weight of the conductor more the force acting vertically downwards and hence greater is the sag value in transmission lines.
(ii) Location of conductor
Sag also depends on the location of conductors.If the conductors are present in area where ice formation takes place,then due the accumulation of ice on the conductor its overall weight increases.This increases the weight of the conductors which in turn increases the value of sag.
(iii) Length of span
Sag is proportional to the square of length of span.Hence, longer the span greater will be the sag provided the tension and weight of the conductor is constant.
(iv) Temperature
The value of sag greatly affected by the temperature.If the temperature is high sag will be more because rise in temperature causes the conductors to expand.Is the temperature is low, the conductor(being metallic) contracts and hence sag is less due to which the tension in the conductor is increases.
(v) Tensile strength
Sag inversely proportional to the tensile strength of the conductor provided the other parameters are constant.
(Vi) Tension
Tension on the conductor is inversely proportional to sag.If the tension is more the conductors are connected very tightly between the tower structure and hence sag is less.On the other hand is tension is less the conductors are connected loosely hands sag is more.
Calculation of Sag in Overhead transmission lines:
(i) When supports are at equal levels
Let us consider a line conductor between two equal height line supports.Line supports are A and B with O as the lowest point as shown in figure.Point O will be the lowest point as two levels are equal lowest point will be at the mid-span.
Let
l = Length of span
w = Weight per unit length of conductor
T = Tension in the conductor.
w = Weight per unit length of conductor
T = Tension in the conductor.
Now consider any point on the conductor.Lets say point 'P'.By considering lowest point O as the origin, let the co-ordinates of point P be x and y. Assuming that the curvature is so small that curved length is equal to its horizontal projection (i.e., OP = x), the two forces acting on the portion OP of the conductor are :
(i) The weight wx of conductor acting at a distance x/2 from O.
(ii) The tension T acting at O.
Equating the moments of above two forces about point O, we get,
T y = w.x * x/2
or
y=Tx²/2T
The maximum dip (sag) is represented by the value of y at either of the supports A and B. At support A, x = l/2 and y = S
Sag, S=w(l/2)²/2T
S=wl²/8T
(ii) When supports are at unequal levels
The difference in level between points of supports and the lowest point on the conductor is called "sag".When transmission lines run on steep inclines as in the case of hilly areas, we generally come across conductors suspended between supports at unequal levels.The shape of the conductor between the supports may be assumed to be a part of the parabola. In this case, the lowest point of the conductor will not lie in the middle of the span.
Consider a conductor suspended between two supports A and B which are at different levels as shown in the following figure.
Let
l = Span length
h = Difference in levels between two supports
x1 = Distance of support at lower level (i.e., A) from O
x2 = Distance of support at higher level (i.e. B) from O
T = Tension in the conductor.
If w is the weight per unit length of the conductor, then,
Sag S1 = wx1²/2T
and Sag S2 = wx2²/2T
Also X1 + X2 = l .........(i)
 |
| If image is not clear on mobile device please download first and view |
X1=l/2-Th/wl
X2=l/2+Th/wl
Having found X1 and X2, values of S1 and S2 can be easily calculated.Effect of wind and ice loading on sag & tension
Till now the expression for sag and tension derived was under normal conditions i.e.. at normal temperature and the weight acting on the conductor was only its own weight. But in cold places there is a ice coating formed on the conductor and also wind pressure nets horizontally on the line conductor. The ice coating on the line conductor increases the total diameter of the conductor and also the weight of the Conductor increases.
The total weight of the conductor i.e., both the conductor weight and the weight of the ice acts vertically downwards whereas the wind force acts horizontally on the conductor. Therefore, the vector sum of horizontal and vertical forces acting on the conductor gives the total force shown in figure.
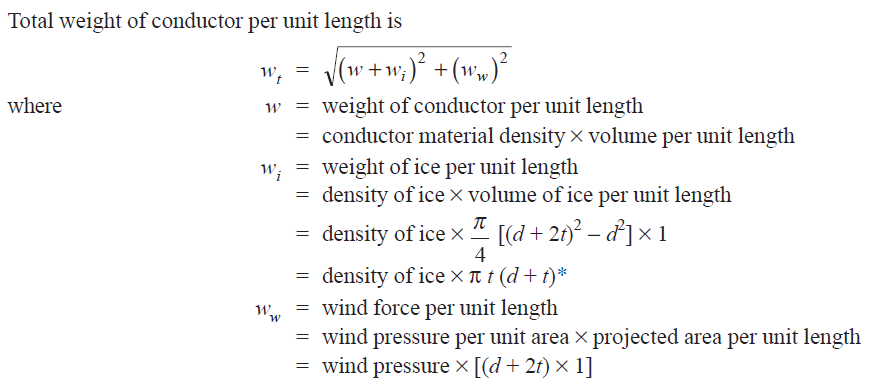 | |
|
When the conductor has wind and ice loading also, the following points may be noted :
(i) The conductor sets itself in a plane at an angle θ to the vertical where
tan θ =Ww/W+Wi
(ii) The sag in the conductor is given by :
S =Wtl²/2T
Hence S represents the slant sag in a direction making an angle θ to the vertical. If no specific mention is made in the problem, then slant slag is calculated by using the above formula.
(iii) The vertical sag = S cos θ.
This is the complete info on sag in transmission line wiki.You can download sag and tension calculation overhead transmission lines as pdf.Share with your friends.
Incoming Searches:
definition of sag in transmission linesag in transmission line pdf
sag in transmission line wiki
sag in transmission line ppt
sag in transmission line formula
what is meant by sag in transmission line
effects of sag in transmission line
transmission line sag and tension




